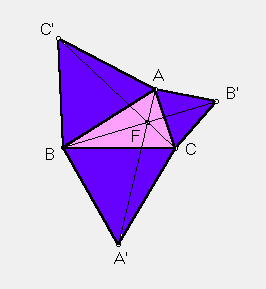
A'BC = the equilateral triangle shown on side BC,
AB'C = the equilateral triangle shown on side CA,
ABC' = the equilateral triangle shown on side AB.
The lines AA', BB', CC' meet in the Fermat point, said to be the first triangle center discovered after ancient Greek times. The great French mathematician Pierre Fermat, posed as a problem the search for a point P for which the sum PA + PB + PC of the distances from P to the vertices is as small as possible. Torricelli proved that the Fermat point, labeled F in the diagram, is the solution if each angle of triangle ABC is less than 120 degrees. Sometimes, F is called the Fermat-Toricelli point.